I start with a translucent cube with a slightly blue tint.

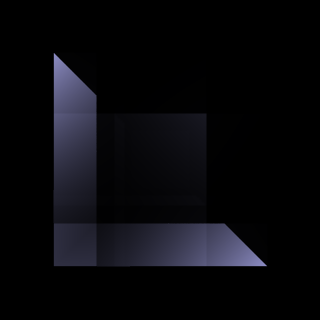
Then I remove material from inside the cube. In this case, I have taken out a sphere whose diameter equals the length of the side of the cube.

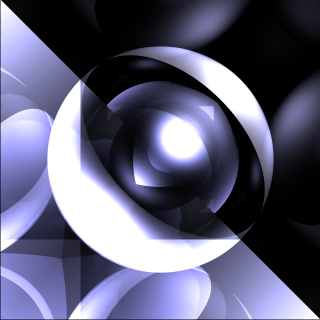
I can also add new objects into the holes, with more material removed from them, too. Here I removed the large cone from the cube, and then added the smaller cone from which the smallest cone was removed. This is one of my favorite scenes.

Here is one of the very first renderings I did, a 2/3 size sphere with a 3/5 size sphere inside it. It is my other favorite scene.

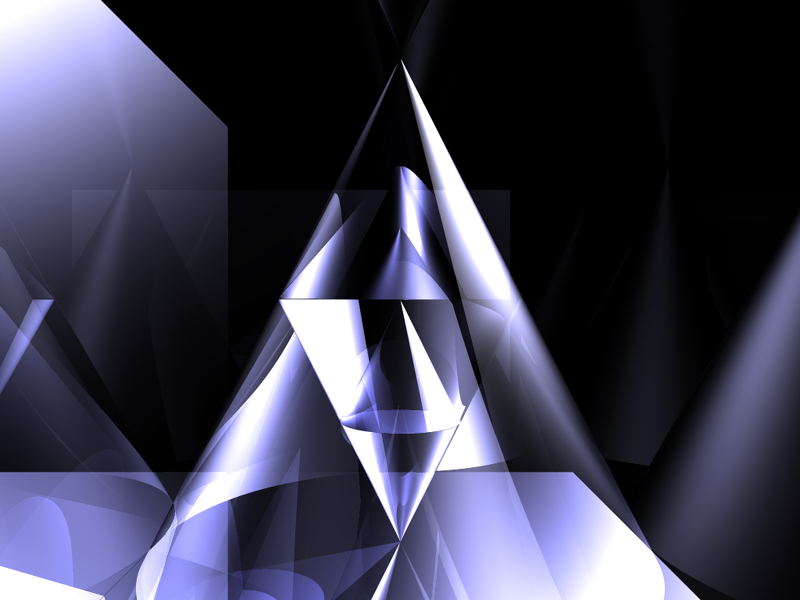
POV-Ray also can also handle index of refraction calculations, as shown below involving a sphere and a couple cones, but I don't think that looks as nice, so I leave that out most of the time.

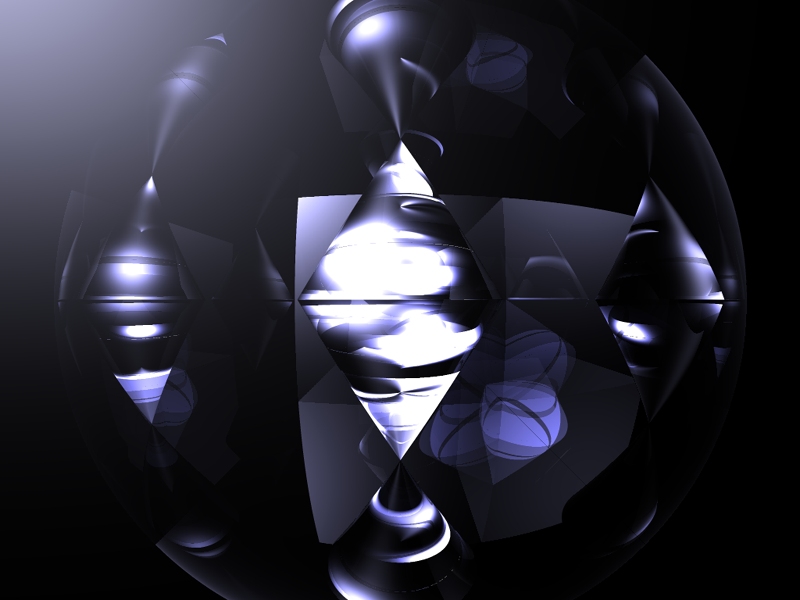
For this image, I used a solid cube, but I had a trigonometric function mapped around the outside, which reflected internally to create all the various geometric objects you can see.

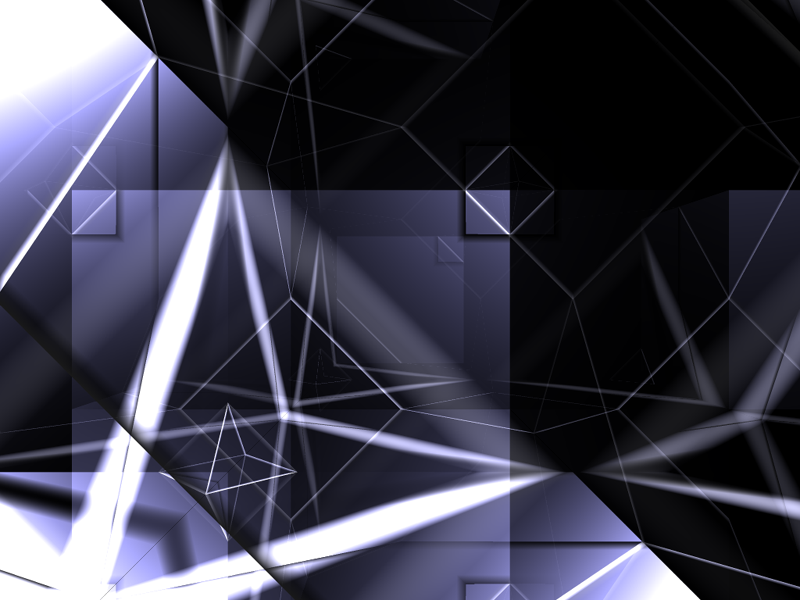
This one represents the unit cell of a face-centered cubic crystal packing geometry.

This image represents the unit cell of a body-centered cubic crystal packing geometry.

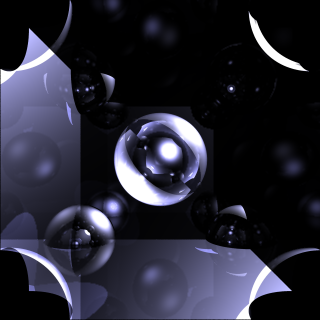
Sometimes I also do objects by themselves without the cube, as in this case.

Here each sphere has twice the radius of the sphere below it. Given an infinite number of spheres like this, they would reach the bottom in the limit. However, I have only rendered 20, although less than that number are discernible.

I had done the original glass renders in MacMegaPov 0.7. When I upgraded to later versions of POV-Ray it started adding a rather unattractive yellow tint in certain cases. Fortunately, MegaPov 0.7 was still on my hard disk at home, and I was able to bring it here. Here is the first new creation I have made with it: 20 randomly distributed spheres of random size.

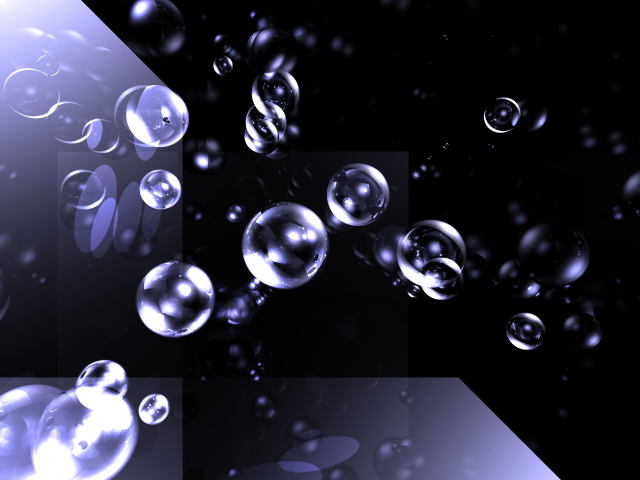
Even with MacMegaPov 0.7, I still wasn't able to achieve the same look I had in the earlier works. However, I realized that I still had the original file that had sparked this whole series, and so I have it once again. Here's one I just did recently after rediscovering the original formula: it is the intersection of three orthogonal cylinders.

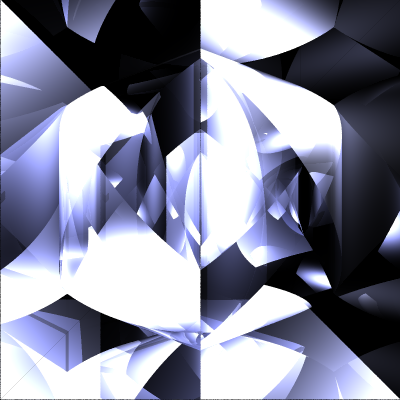
This one consists of an inverted pyramid of three cylinders.